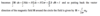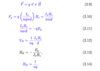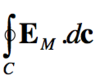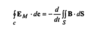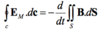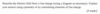Questions & General Notes Flashcards
(61 cards)
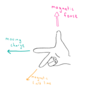
Sketch the electric field around an electric dipole.
Include equipotential lines and the direction of the field.
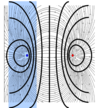
Sketch the Electric Flux Density around a sharp conducting rod, raised to a potential V.
Explain why this is suitable for a lightning conductor.
Taking part of the conductor as a sphere with radius a then the field at the surface of the conductor is…





Solve using boundary conditions.

Important to remember the how the components relate for two different dielectrics.

How would you solve this problem?

First, calculate the LHS of Gauss’s law, which looks at the surface area. If the surface is spherically symmetrical, the flux is leaving radially and the magnitude is uniform, so D can be considered a constant and factored out of the integral. The resulting integral simply calculates the total surface area.
Next, you look at the RHS of the equation and calculate the integral of the charge density over the volume v. Since the charge density is constant it can be factored out of the equation.
The two results are equated to eachother to find D.
The final expression needs to include the vector direction. This is done by multiplying D by the unit vector of r. You also need to remember to include the radial direction, which in this case is negative.
To find the electric flux density outside of r, the calculations need to adjusted. RHS of the equation stays the same, but for the LHS r becomes a, since the spherical cloud is limited by radius a, it doesn’t go any further…

If you need to apply Gauss’s law to a sphere and there is spherical symmetry, what can you assume about the equation describing the total flux leaving the Gaussian surface?
Since the sphere is symmetrical all around, the flux leaves the surface radially. As a result, the dot product of D and dS is essentially equal to the product of the magnitude of the two. With the magnitude being uniform, D can be taken out of the integral as a constant for the fixed radius (if the magnitude of D changed this wouldn’t be possible).

The figure shows the current carrying wire and an element dl somewhere along the wire at a distance r from the point at which the magnetic flux density B is required. The Biot Savart law gives the direction (out of the page in this case) from the cross product in the equation. It also gives the magnitude of dB due to just that small element of current I.

Magnetic Field around a straight current carrying wire, coming out of the page.



What do the dot product and cross product represent?
The dot product of two vectors A and B is the length of the projection of A onto B multiplied by the length of B.
The magnitude of the cross product is the area of a parallelogram (four-sided rectangular figure) with two sides based on vectors A and B. The direction of the cross product is orthogonal to the plane containing this parallelogram.
In other words, the cross product is a geometric construction that gives a vector perpendicular to two other vectors and the resultant vector has a length equal to the area of the parallelogram.
What do you do in this case?

You have to equate the x component to zero.
Using Gauss’s law, what is the flux through a spherical shell of radius R with

When you’re determining the electric field outside of a shell of uniformly distributed charge, what can you immediately assume?
That the charge enclosed is equal to the charge coming from the shell.
Thus when taking the Gaussian surface greater than the radius of charged shell, the shell can be essentially considered a point charge.
How do you solve this?

In the first line they determined that the integral of D.ds could be written as 4πr2 .

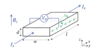
What is the total flux leaving the cube?
Anything you need to remember?

That in a uniform field, objects will not have a net flux leaving it.
What should you not forget when using electric field equations or calculating them using gauss’s?
the unit vector:
ar
What is the potential difference?


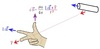
Right hand rule for biot-savart.
middle finger is the unit vector
index is Idl
thumb is the direction of dB

When asked to draw a vector field, what do you need to remember?
That’s it’s a field, and thus implies multiple vectors…

What do you need to remember about this question?
Although marks were awarded for if only x and y were included, z should be written down as well.

What is a expression for force that you need to remember for the exam?
As it isn’t given in the formulaa sheet.
For a length of wire l this becomes: F = I*L*uLx B
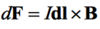
What did you learn about this question?
If no electric field is defined, and they only talk about electric fields, do not use the full lorentz force eq.
Also, that you can use: dF = IdLxB

RH rule for magnetic field, current and force.