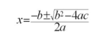Random Flashcards
(150 cards)
1
Q
What are the properties of a 30 - 60 - 90 triangle?
A
base = x
height = x * 3 squared
hypotenuse = 2x
2
Q
The fraternity must choose 3 senior members and 2 junior members for a conference. If the fraternity has 6 senior members and 5 junior members, how many different delegations are possible?
A
- Senior members: 6! / (3!3!) = 20
- Junior members: 5! / (2!3!) = 10
- Total possible delegations: 20 * 10 = 200
3
Q
What is a perpendicular bisector?
A
A line which cuts a line segment into two equal parts at 90°.
4
Q
Circle. An central angle of 120 correspods to an inscribed angle of…?
A
60
5
Q
Company K and R have 17 directors in total. How many people are directors of both companies if Company K has 8 directors and Company R has 12 directors?
A
20 - 17 = 3
6
Q
xy = 1
Does this mean that x = y = 1?
A
Not necessary. It might also be tru that x=1/5 and y=5.
7
Q
Square root of 25 =
A
5
or
-5
8
Q
What is the median of 16 terms, arranged in increasing order?
A
The median = the mean of the 8th and 9th term
9
Q
[Original] [Percent] [Change]
Percent =
A
(Change / Original) * 100
10
Q
Can we solve
2x - 17 + c/6 = 7y
if we know that 16x = 56y + 23
Explain.
A
Yes, we can. We have 2 equaions with 3 variables, but with proportions.
16x - 56y = 23 (1)
2x - 7y = 17 - c/6 (multiply all values by 8)
16x - 56y = 136 - 8c/6 (2)
Now we know that
23 = 136 - 8c/6
We can solve it.
11
Q
When all the dimensions of a three-dimensional object are changed by a factor of 2, volume changes by what factor?
A
Volume changes by a factor of 8.
12
Q
What is the price of 5 donuts and 3 bagels, if 10 donuts and 6 bagels = 12.90$
A
Its 6.45$
13
Q
In a vertex equation whats the meaning of each part?
h = -16(t - 3)2 + 150
A
- 3 = the time needed to reach the maximum height
- 150 = the maximum height
- t = time passed
14
Q
(x - y)(x - y) =
A
(x - y)2 = x2 -2xy + y2
15
Q
What are the 2 formulas for overlapping sets problems?
A
- Total = A + B + C - (sum of 2 group overlaps) + (all three) + (neither)
- Total = A + B + C - (sum of exactly 2 overlaps) - 2(all three) + (neither)
16
Q
What is the value of Pi?
A
3.14159
17
Q
Circle. An inscribed angle of 40 correspods to an central angle of…?
A
80
18
Q
|12-5| + |6| - |-8| = ?
A
5
19
Q
Circle. Area formula
A
Area = radius2 * pi
20
Q
x2 + 2xy + y2 =
A
(x + y)2 = (x + y)(x + y)
21
Q
What is the digit number of 3429 + 9414?
A
0
Check OG PS 163
22
Q
x/y = 1/4
Does this mean that x = 1 and y = 4?
A
Not necessary. It might be that x = 25 and y = 100 or other analog.
23
Q
4.56 * 107 = ?
A
45600000
24
Q
Speed Time Distance
Time =
A
Distance / Speed
25
What is **the range?**
The range is the difference between the largest and the smallest number.
26
x2 + y2 =
(x - y)(x + y)
27
12 machines, each working at the same constant rate, together can complete a job in 84 hours. How many more machines will be needed to complete the job in 48 hours?
**9**
First we have to find out the rate of one machnie:
12/r = 1/84, r = 1008
It takes 1008 hours for one machine alone to complete the job. Now we have to find out the number *x* of machines needed to complete the job in 48 hours:
x/1008 = 1/48, x = 21
21 - 12 = 9 additional machines needed
28
What is the diagonal of a rectangular with sides 2 and 3?
diagonal2 = 22 + 32 = 12
diagonal = root of 12
29
How can we find the average speed of a boy that walked the same distance twice, at two different rates?
Average speed = total distance / total time
30
What is the weighted average formula?
Weighted average = (component 1)(weighting 1) + (component 2)(weighting 2)
Example:
100 \* 0.80 + 80 \* 0.20 = 80 + 16 = 96
31
What is the number of possible ways to arrange letters
## Footnote
**AJJKLO**
6! / 2! = 360
32
How many equations do we need to solve for 4 variables?
4
33
Speed Time Distance
**Speed =**
Distance / Time
34
How is **Standard Deviation** calculated?
1. We have to find out the mean.
2. Each element is subtracted from the mean. The absolute value of each result is added up and divided by the number of results.
35
What is the sum of 14 first integers?
The formula for the sum of n first integers is (n (n + 1)) /2, so
(14 (14 + 1)) / 2 = 7 \* 15 = 105
36
What is the quadratic formula?
37
Explain two formulas for 2 successive % change?
1. Original value \* ( 1 (+ or -) x/100) \* ( 1 (+ or -) y/100) = New value
2. (+ or -) x (+ or -) y (+ or -) xy/100 = total percent change
x - first percent change
y - second percent change
Depending if change is positive or negative, so are the signs next to x or y.
If x or y is negative, then xy/100 is negative too.
38
When an integer is divided by 10, what is the reminder? What about the case when it is divided by 100?
When an integer is divided by 10 the remainder = units digit value of that number.
For example when 123 is divided by 10, the remainder = 3.
When an integer is divided by 100, the remainder = the value of its tens and units digits.
For example when 123 is divided by 100, the remainder = 23.
39
What is the length of the side of a square if the diagonal is = 16 ?
16 / root of 2
40
We have a coorfinate plane with the following equation:
y = (x - c) (x + a)
Which value can be the x intercept?
When the line intercepts x, y = 0, x = c and x = -a
41
If the cost of a certain house in 1983 was 300 percent of its cost in 1970, by what percent did the cost increase?
**200%**
Use smart numbers. For example in 1970 the cost was 100$. In 1983 it was 300$ (100$ \* 300% = 100 \* 3 = 300$). The change is 200$. Percent change 200/100 = 2 = 200%.
42
What is the compounded interest rate formula?
Total = Principal(1 + r/n)nt
r - interest rate
n - number of times compounded per year
t - nr of years
43
How many even integers are between 12 and 24? What is the formula for solving?
**Formula:** (Last - First) / increment (multiplier) + 1
**Solving:** (24 - 12) / 2 + 1 = 7
44
x2 - 2xy + y2
(x - y)(x - y) = (x - y)2
45
0 = 1 + 2xy + y2 x2
What is the value of *xy*?
1 + 2xy + y2 x2 = 0
(xy + 1)2 = 0
xy + 1 = 0
**xy = -1**
46
What is the sum of all integers from 75 to 150 inclusive?
Solving:
1. We need to find the average: (75 + 150) / 2 = 112.5
2. We need to find the number of terms: (150 - 75) + 1 = 76
3. The sum = average \* # of terms: 112.5 \* 76 =
47
(x + y)(x + y) =
(x + y)2 = x2 + 2xy + y2
48
If *p* is the product of integers from 1 to 25, inclusive, what is the greatest integer *x* for which 2x is a factor of *p.*
x = 22
It is basicaly how many 2s fit into 25!.
(Check OG PS 116)
49
What is the median of a set of numbers?
If the number of elements in the set is even, the median equals to the average of the middle two elements.
If the number of elements in the set is odd, the median equals to the middle ellement.
Condition: the elements are arranged in increasing or decreasing order.
50
Of 11 terms, arranged in increasing order, which one is the median?
The 6th
51
What is the diagonal of a cube with a side of 10?
10 \* root of 3
52
What is a hemisphere?
A hemisphere is a half of a sphere.
53
How many multiples of 7 are there between 100 and 150?
We can solve this 2 ways:
**1. Using the formula (Last - First) / increment + 1**
Last multiply of 7 is 147, first is 105. (147 - 105) / 7 + 1 = 7
**2. By counting**
105 112 119 126 133 140 147
54
What is the aferage formula?
Average = sum / # of terms
55
If |x-2| = |2x-3|, what are the possible value for x?
Each absolute value expression has 2 algebraic cases – positive or negative. So we have 4 cases overall: positive/positive, positive/negative, negative/positive and negative/negative.
The first and last cases deliver the same results. The same with the 2nd and 3rd. So we have 2 cases:
When there is same sign:
(x-2) = (2x-3) ≫ 1=x
When signs are different:
(x-2) = -(2x-3) ≫ 5/3=x
56
Square root of 400 =
20
or
-20
57
58
If a and b are positive integers,
and a/b = 2.86
what are the possible divisors of a?
13 and 11
a/b = 2.86
a/b = 286/100
a/b = 143/50
a \* 50 = b \* 143
b is divisible by 2, 5, 25 and 50
a is divisible by 11 and 13
59
How many equations do we need to solve for 3 variables?
3
60
**[Work] [Rate] [Time]**
Work = ?
Rate = ?
Time = ?
Work = rate \* time
Rate = work / time
Time = work / rate
61
Is *y* an integer?
*y2 *is an integer
Cannot be determined.
If y = 3, then 32 = 9 and 9 is an integer.
If y = square root of 4, then (square root of 4)2 is an integer too.
62
Factor out
21z + 21z-1
21z-1(1 + 21) = 21z-1(22)
63
What is the mean of a set of numbers?
The average of a set.
64
What is the probability that 2 6-sided cubes will yield a sum greater than 10?
1. There are 36 posibilities. 6 \* 6
2. To have 11 or 12 we need either a 5 + 6, 6 + 5 or 6 + 6.
_6 + 6 does not repeat_
Thuse, its 3 / 36.
65
Speed Time Distance
**Distance =**
Time \* Speed
66
What is the reciprocal of 1/12?
12/1
67
If a triangle is divided by a perpendicular to its hypotenuse and forms 2 triangles, what are the properties of these 2 triangles?
These 2 triangles have same properties and same proportions.
http://gmatclub.com/forum/if-arc-pqr-above-is-a-semicircle-what-is-the-length-of-20274.html?kudos=1
68
521 \* 411 = 10n \*2
What is the value of *n*?
Explain the process.
511 \* 510 \* 411 = 2 \* 10n
2011 \* 510
2010 \* 510 \* 20
10010 \* 10 \* 2
(102)10 \* 10 \* 2
1021 \* 2 = 10n \* 2
n = 21
69
Of 23 integers, arranged in increasing order, which one is the median?
The 12th
70
What is the digit number of 169 + 3625
2
Check OG PS 163
71
What are the roots of a quadratic equation?
The roots are the results.
| (x +- root1)(x+-root2)
72
Coordinate
A negative slope =
A decreasing line ( passing through quadrants 2 and 4)
73
74
Each member of a team received *x* tools, *y* pieces of paper and *z* pencils. How many team members are in the team if the total number of objects distributed are 18 tools, 27 pieces of paper and 36 pens?
Cannot be found. Because there can be 1 team member that received all the objects, or 9 team members and each received 2 tools, 3 papers and 4 pens.
75
w = 1
x = 5
y = 6
76
What is the difference between
(-10)20 and -(10)20
(-10)20 = 1020
-(10)20 = -1 \* 1020
77
31/3 is more ore less than 1?
More.
Root of any integer grater than 1 is bigger than 1.
78
a = -0.3
Put the next elements in increasing order:
a; a2; a3
a \< a3 \< a2
because:
-0.3 < -0.027 < 0.09
79
What is a subset?
When the elements of set X are in set Y, X is a subset of Y.
80
The average score of the girls and boys at a certain college is 80. Is the average score of girls greater than 85 if the average score of boys is less than 75? Solve and explain.
We cant find out because we dont know the number of boys and of girls.
81
Express the ratio of X to Z.
X/Z
82
What is the diagonal of a square with side = 10?
10 \* root of 2
83
How many integers are between 201 and 300 inclusive?
**100**
84
A master makes a box with a capacity of 12 cubic feet. Then he makes a similar box, but twice as wide, twice as long and twice as high. What would be the capacity of the second box? Explain.
When all the dimensions of a three-dimensional object are changed by a factor of 2, the capacity (volume) changes by a factor of 8.
12 \* 8 = 96
85
1! = ?
2! = ?
3! = ?
4! = ?
5! = ?
6! = ?
1! = 1
2! = 2
3! = 6
4! = 24
5! = 120
6! = 720
86
x = y
x = 1 - 3t
y = 2t - 1
Solve for t.
We know that x = y, this means
1 - 3t = 2t - 1
2 = 5t
t = 2/5
87
Square root of 289 =
7
or
-7
88
Circle. Circumference formula
Circumference = diameter \* pi
89
Of the 50 reserchers, 40% will be assigned to team A and 60% to team B. However 70% prefer team A and 30% prefer team B. What is the lowest possible number of reserchers who will not be assigned to the team they prefer?
**15**
20 out of 35 (who prefer team A) will be assigned to team A. It means 15 will have to go to the other team.
Common mistake is double counting the ones that dont prefer team A and the ones that dont prefer team B.
90
What is the formula for calculating the sum of an evenly spaced set?
Sum = average \* # of terms
91
What is the sum for the first 26 integers?
The formula for the sum of *n* first integers is (n (n + 1)) /2, so
(26 (26 + 1)) / 2 = 13 \* 27 = 351
92
Can we define the value of y/x if x2 = y2?
No.
If the squares of x and y are equal it doesnt mean their square roots are equal. For example if x2 = 36, its square root can be equal with either 6 or -6. The same case applies to y.
93
How many integers are there from 14 to 765, inclusive? What is the formula of calculation?
**Formula:** (Last - First) + 1
**Solving:** (765 - 14) + 1 = 752
94
What are the properties of a **45 - 45 - 90** triangle?
45 side = x
90 side = x \* 2 squared
95
When we have to find the smallest possible number in a list of unknowns, what should we assume?
We should assume that the other numbers are as big as possible.
96
What is the number of possible ways to arrange letters
## Footnote
**ABC**
3! = 6
97
What is the quotient and the reminder of the division of a smaller integer by a bigger integer?
The quotient = 0
The reminder = the smaller integer
98
**[Original] [Percent] [Change]**
Original =
Original = Change \* (100/Percent)
99
What do word marks "or" "and" mean in probability problems?
Or = add
And = multiply
100
2.47 \* 10-3 = ?
0.00247
101
13 / 0 = ?
Division by 0 is not defined.
102
Is m odd, if m/2 is not an even integer?
No.
Because *m* can satisfy this condition if it was 10 or 3 or any other number.
103
What is a *intersection* of sets?
The set of elements that are in both X and Y sets.
104
What is the reciprocal of 3/5?
5/3
105
3150 multiplied by *y* must be a perfect square of a number. What is the minimum possible value of y?
For a number to be a perfect square it must have a even number of its prime factors.
3150 = 52 \* 32 \* 7 \* 2
We need a 7 and a 2 for 3150 to be a perfect square.
The answer is: 14
106
Work Rate Time
1. When more workers are working together, each at his own rate, how can we find out how much of a job they can do working together?
2. What about when one worker is undoing the work of the other one?
1. Add the rates.
2. Subtract the rates.
107
Express "the 12th root of w."
w1/12
108
What is the Area of a rhombus?
(Diagonal1 \* Diagonal2) / 2
109
Express the ratio of 5 to 6 in 3 ways.
1. 5 to 6
2. 5:6
3. 5/6
110
Is 0 positive or negative?
0 is neither positive nor negative.
111
Why is the formula **P(A) + P(Not A) = 1** helpful?
Because in probability problems when it is hard and time consuming to find the probability of smth to happen, it is easier to find out the probability of the event not happening. By subtracting the result from 1 we find out the probability we needed in first place.
112
What is the range of possible remainders when X is divided by Y?
from 0 to y - 1
113
If the triangle is isoscel, then its a 45 45 90 triangle.
True/False? Why?
No, its not.
And isoscel triangle can have very different side lengths.
50 50 80
75 75 30
etc.
114
Lenas grade is in the 80th percentile out of 120 grades in her class.
## Footnote
**What does this mean?**
This means that 80% out of 120 students have grades lower than Lenas.
115
John decided to make a sandwich. He has 3 types of bread, 6 types of ingredients and 4 types of sauce.
If John decides to make a sandwich with 3 ingredients, how many different types of sandwiches can he make?
Types of bread \* possible ingredients \* types of sauce
We have 3 types of bread
Possible ingredients: 6! / (3!3!) = 20
Types of sauce: 4
3 \* 20 \* 4 = 240
116
14112 multiplied by y must be a perfect square of a number. What is the minimum possible value of y?
For a number to be a perfect square it must have a even number of its prime factors.
14112 = 7 \* 22 \* 32 \* 3 \* 2
We need a 7, a 2 and a 3 for 14112 to be a perfect square.
The answer is: 42
117
**Express this in 2 equations:**
The present ratio of students to teachers at a certain school is 30 to 1. If the student enrollment were to increase by 50 students and the number of teachers were to increase by 5, the ratio of students to teachers would then be 25 to 1. What is the present number of teachers?
1. 30/1 = s/t
2. s + 50 / t + 5 = 25/1
118
(x - y)2 =
(x - y)(x - y) = x2 -2xy + y2
119
A pod of 8 whales always swim in the same way: 3 white whales swim on the right and 5 blue whales swim on the right side of the group. In how many different arrangements can the whales swim\>
We have two independent groups: 3! and 5!
3! = 6
5! = 120
6 \* 120 = 720
120
Each of the 10 nest contains the same number of eggs. What is the standard deviation?
The standard deviation is 0.
121
A club will send 3 representatives to the national conference. If the local club has 8 members, how many different groups of representatives could the club send?
8! / (5!3!) = 8 \* 7 = 56
122
If you know that:
X is the midpoint of AC
Y is the midpoint ob BC
S is the midpoint of YC
R is the midpoint of XC
What can you tell about the properties of this triangle?
1. ABC = 2ACY = 4XYC = 8CSX = 16CRS
2. 2ABY = ABC
3. ABY = ACY
4. CXY = AXY
123
**[Original] [Percent] [Change]**
Change =
Original \* (Percent / 100)
124
Square root of 169 =
13
or
-13
125
(x + y)2 =
(x + y)(x + y) = x2 + 2xy + y2
126
Express "the 5th root of w".
w1/5
127
What is the sum of interior angles of any polygon?
Sum = 180(n - 2)
n = the number of angles
128
What is the "altitude" of a triangle?
The altitude of a triangle is the perpendicular from the base to the opposite vertex.
129
The hypotenuse of a right triangle = 10 cm. The area of the triangle = 25. What is the perimeter of the triangle?
x and y will be the unknown sides of the triangle. Since we know the length of one side (10) to find out the perimeter we need to find out the sum of the unknown sides.
x2 + y2 =102 (pythagora + hypotenuse)
1/2xy = 25 xy = 50 (area)
(x + y)2 = x2 +2xy + y2 = 100 + 100 = 200
x + y = square root of 200
130
Square root of 169 =
13
or
-13
131
What is the formula for finding the average of an evenly spaced set?
(First number + Last number) / 2
132
To solve for x, what shall be done next?
1. Multiply both sides by 2.
2. Divide both sides by 2.
3. Add x to both sides.
1. Multiply both sides by 2.
133
Find out the integer **n:**
n (n + 1) = 6
**Impossible.**
n2 + n - 6 = 0
(n + 3)(n - 2) = 0
n = 2 or n = -3
It is not possible to find out.
134
What is the formula for the sum of the first *n* integers?
= (n (n + 1)) / 2
135
Is x/y an integer if every prime factor of y is also a prime factor of x?
No.
For example if y = 6 (2 \* 3) and x = 18 (2 \* 3 \* 3), x/y will be an integer,
However, if y = 8 (2 \* 2 \* 2) and x = 18 (2 \* 3 \* 3), x/y will not be an integer.
136
0! = ?
1
137
Express "the cube root of w".
w1/3
138
If an inscribed triangles hypotenuse is the diameter of the circle, what kind of triangle it is?
Its a right triangle.
139
For a number to be a perfect square, the number of its every prime factor must be _____ .
Even.
140
If *x* and *y* are non zero integers, which one of the following values will always be negative? Explain why.
- (x-2y)
- (x-3y)
- (x-2y) will always be negative, because whether x and y will be positive or negative, the value in the brackets will become positive. The minus sign in fron of the brackets makes the value negative.
- (x-3y) can be either positive or negative. For example if y is even, the final value will be negative. If y is odd and x is negative, the final value will be positive.
141
How is calculated the area of a equilateral triangle?
Method 1:
(base \* height) / 2
Method 2:
1. Divide it into two 30 - 60 - 90 triangle
2. Find out the base and the height of the 30 - 60 - 90 triangle
3. Calculate: base \* height (of the 30 - 60 - 90 triangle)
142
What is a *union* of sets?
A union a set which includes all the elements of two sets.
143
What is the formula of the reminder of y/x?
y = x \* quotient + reminder
This formula can also be written as:
y/x = quotient + reminder/x
144
Circle. An inscribed angle of 25 correspods to an central angle of...?
50
145
Solve
(56 - 46) =
(56 - 46) = (53 + 43)(53 - 43) = (125 + 64) \* (125 - 64) = 189 \* 61
146
A number is divisible by 3 if...
If the sum of all its digits is divisible by 3.
147
A number is divisible by 6 if...
If the units digits is even and the sum of all the numbers digits is divisible by. Rule for divisibility by 2 + rule for divisibility by 3.
148
A number is divisible by 7 if..
Double the units digits subtracted from the number / 10 - decimals is divisible by 7, then the nr is divisible by 7.
Example: 553 is divisible by 7 because:
3 \* 2 = 6 (units digits doubled)
55 (553/10 - 0.3 = 55) - 6 = 49 (49 is divisible by 7)
149
If *a* divided by 75, leaves a remainder of 3, what remainder will have the division of *a* by 5?
The remainder will be 3. *a* divided by all the factors of 75 will leave a remainder of 3. Except 3 ofc, then the remainder will be 0.
150
If *a* divided by 75, leaves a remainder of 5, what remainder will have the division of *a* by 3?
By the usual approach, when a number divided leaves a certain remainder, then the same number divided by any factor of the divisor leaves the same remainder. BUT the case when the divisor is smaller than the remainder, in which case we subtract the smaller divisor from the bigger remainder. In our certain case we have 5 - 3 = 2. *a* divided by 3 will leave a remainder of 2.
