SS - Periodicity, crystal systems and unit cells Flashcards
Define a lattice
An infinite array of points where each point has identical surroundings
Define a unit cell
A building block which when repeated in all directions gives the lattice
Define a lattice point
A single point within a lattice. The separation between lattice points is a, and knowledge of one point and a can build the whole lattice
Define a motif
Otherwise known as a basis. A motif/basis represents one or more atoms, ie a molecule or a protein. When a motif is placed on a lattice, a crystal structure is formed
Describe how to count atoms in a 2D representation
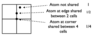
Describe how to count atoms in a 3D representation
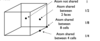
State the 4 types of unit cells
- Primitive (P)
- Body centred (l)
- Face centred (F)
- C centred (C)
Draw a primitive unit cell
Draw a body centred unit cell
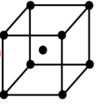
Draw a face centred unit cell
Draw a C centred unit cell
How many lattice points does a primitive unit cell have
1
How many lattice points does a body centred unit cell have
2
How many lattice points does a face centred unit cell have
4
How many lattice points does a C centred unit cell have
2
Draw a unit cell and label the parameters
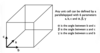
Define bravais lattices
Combinations of the 7 unit cell shapes and 4 possible lattices give 14 Bravais laticces
State the 7 unit cell shapes
- Cubic
- Orthorhombic
- Tetragonal
- Triclinic
- Rhombohedral
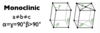
- Monoclinic
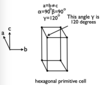
- Hexagonal
Give the parameters for a cubic unit cell
Give the parameters for an orthorhombic unit cell
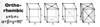
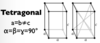
Give the parameters for a tetragonal unit cell
Give the parameters for a triclinic unit cell
Give the parameters for a rhombohedral unit cell
Give the parameters for a monoclinic unit cell
Give the parameters for a hexagonal unit cell
Describe a crystal structure in terms of Bravais Lattices
Crystal structure = bravais lattice + basis
Give the parameters of the hexagonal primitive cell
Define the coordination number of an atom and give the coordination numbers for each type of cubic unit cell
The number of atoms bonded to the atom in question gives its coordination number. Ie the number of nearest neighbours.
Describe miller indeces
- Miller indices are used to describe planes in crystals, and to distinguish between different surfaces expressed by a crystal.
- Planes diffract X-ray radiation which can be used to determine crystal structures.
- A miller index takes the form (h k l) where h, k and l are integers.
- The plane (h k l) intercepts the a, b and c axis at a/h, b/k, c/l.
- The separation between planes is referred to as dhkl.
- Note: -2 = 2 bar
Give the volume of a cubic unit cell in terms of a
V = a3
State the equation for density
p = M/V
