Lecture 1: Utility Maximization Flashcards
Utility Maximization
Consumers choose the best bundle of goods (that maximize utility) among the ones that are affordable.
Two Primary Utility Maximization Approaches
- The decision maker’s tastes as summarized in her “preference relation”
- Assumptions of Individual Choice Behavior
The decision maker’s tastes as summarized in her “preference relation”
The theory is developed by imposing rationality axiom on the decision-makers’ preference and then analyzing the consequences of these preferences for her choice behavior in some set of X alternatives called the consumption set.
Individual’s choice behavior
Proceeds by making assumptions directly concerning this behavior
Two classic assumptions of individual choice behavior
- (WARP) weak axiom of revealed preferences
- (SARP) Strong Axiom of Revealed Preferences
Assumptions of preferences
- Time and space are fixed
- No negatives goods
*
Why are time and space fixed?
- Commodities in different time and spaces are considered different goods (umbrella in Oklahoma v Seattle at various times of the year).
The consumption set is denoted as ___
We assumed that x is ___ and ___
Closed and Convex
A closed set is ___
a set that includes its boundary points.
x is closed if ____
every convergent sequence in the set X converges to a point in x.
Physical Constraints of Consumption Goods
- Must be consumed in integer amounts
- Fixed space and time for goods
- Consumption set reflecting survival needs
The simplest consumption set
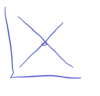
Example of non-convex set
Example of convex set
Strict preference relation
known as the weak preference relation
The indifference relation
Standard properties used to order the set of bundles
Understanding the complete preference assumption
The consumer must be able to make a choice between the bundles of goods. Indecision will make it impossible to model choices.
Understanding the transitivity assumption
this is necessary for rationality and consistency of the consumer’s preferences.
Preference relation is rational if
it is complete and transitive
The important part is that: rationality of >~ implies both > and ~ is transitive
Continuity
Why do we need the continuity assumption
It is necessary to rule out certain discontinuities. So we can assume the smoothness of consumer behavior.
If we are working with continuous functions then we can’t find solutions.
Most importantly: if y is strictly preferred to z and if x is a bundle close enough to y, then x must be strictly preferred to z.
Utility function
a function, u: x -> R, representing preference relation
>~ (math def.)
if for all x,
Level curves in utility functions
These are indifference curves.
They represent different combinations of goods that provide the same utility.
> (math def.)
Weak Monotonicity
~> satisfies if
Thus, there can be cases where vectors can be equal rather than strictly greater.
if x in X, then x >> y then x >y
ex: (3,2) >> (2,2) -> x ~> y
Strict Monotonicity
Strong monotonicity says that at least as much of every good and strictly more of some good is strictly better.
Euclidian distance between two points
Try a problem until you can do it in your head
Local Nonsatitation
A weaker notion that monotonicity
Graph of monotonicity and local nonsatiation
Convexity
Show convexity graphically
Strict convexity
Convexity implies that an agent prefers ___
How does this translate graphically between strict convexity and normal convexity
averages to extremes
Convexity is a generalization of ___
the neoclassical assumption of diminishing marginal rates of substitution.
Existence of a utility function: Supposes preferences are ___
- complete,
- reflexive,
- transitive,
- continuous,
- and strongly monotonic.
If there exists a utility function, then there exists ___
