Numerical Integration Flashcards by Hollie Pilkington | Brainscape

Q

What is the main question in this chapter?

A

How do we calculate integrals numerically?

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

What is a definite integral.

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

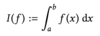
What is a quadrature formula?

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

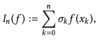
What are x₀, …. x_n and σ₀, … ,σ_nin the following?

A

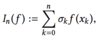
x₀, …. x_nare a set of nodes
σ₀, … ,σ_nare a set of corresponding weights

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

What are the nodes also known as in the following?

A

Quadrative points

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

What is the quadrature formula for the trapezium rule?

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

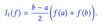
How can you derive quadrative formulas?

A

By integrating polynomials interpolants at equally spaced points.

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

What is the basic form of the interpolating polynomial in Lagrange form?

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

By integrating the following p_n(x) what is the quadrative formula you get?

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

If the nodes are equidistant what is the following formula called?

A

Newton-Cotes formula

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

When is the Newton-Cotes formula called closed?

A

If x₀=aand x_n=b

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

Prove the trapezium with using the closed Newton-Cotes formula.

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

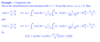
What is the theorem about the Newton-Cotes formula error bound?

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

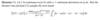
Prove the following theorem.

A

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

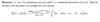
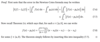
Why don’t Newton-Cotes formulae converge as n ➝ ∞?

A

Because they are based on polynomials interpolation at equally-spaced nodes

How well did you know this?

1

Not at all

2

3

4

5

Perfectly

Q

What is the idea with composite Newton-Cotes?

A

Divide [a,b] into m subintervals [x_i-1,x_i] of equal length h = (b-a)/h and use a N-C formula with small n on each interval.

Q

What is the composite trapezium rule?

A

Q

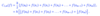
What is the overal error for the composite trapezium rule?

A

Q

What is the trapezium rule exact for?

A

All lienar polynomials

Q

Define degree of exactness.

A

Q

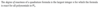
How do you check for degree of exactness?

A

Check the standard basis 1, x, x², … ,xⁿ

Q

What is the Newton Cote’s formula for n=2 called?

A

Simpson’s rule

Q

What is simpsons rule?

A

Q

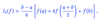
What is the main idea in Gaussina Quadrative?

A

Q

Finish the following lemma.

A

Q

Prove the following lemma.

A

Q

What is the Gaussian quadrature theorem?

A

Q

What is the difference between Newton-Cotes and Gaussian quadrature?

A

Both are based on integrating an interpolating polynomial, but for Gaussian the nodes are the roots of an orthogonal polynomial, rather than equally spaced points.

Q

Prove the following theorem.

A

Q

What is the Mean value theorem for integrals?

A

Q

Prove the following theorem.

A

Q

What is the Error estimate for Gaussian quadrature theorem?

A

Q

Prove the following theorem.

A

Q

A