Lecture 9- Can we predict population dynamics?

Question 1

What is this equation?

Answer 1

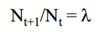
• finite rate of increase
• if lamda =1-no change

larger than 1-increase in population

smaller than 1-decrease in population

• values can be 0 to 1 to infinity (theoretically)
• get it from two points on graph at different times

Question 2

What is this equation?

Answer 2

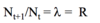
• fundamental net reproductive rate
• if all the resources available and lamda constatnt over time we call it R

R= maximum rate at which the population will increase in that environment if resources unlimited

• only when lambda constant it is R
• can get R from= sum of percentage surviving x fecundity /100

Question 3

If we assume continuous growth what can we do with the growth graph?

Answer 3

• plot log of abundance aginst time
• becomes straight line
• the slope of the line= R

Question 4

What is this equation?

Answer 4

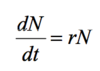
• get it from differentiating the exponential growth equation
• use it for continuous growth
• when we know continuously what is happening
• differential equation

r= intrinsic rate of natural increase in that habitat

Question 5

What is this equation?

Answer 5

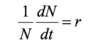
• rearranged version of dN/dt=rN
• per capita rate of increase while in exponential growth

Question 6

Which equation would we use for discrete growth ie. ehrn we know what is happening only at some times and not in between?

Answer 6

Question 7

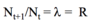
What is a famous saying by Thomas Robert Malthus about demand for resources?

Answer 7

• at some point demand for resources will exceed demand (e.g. food)
• populations cannot continue to grow exponentially

Question 8

What are the positive controls for population size?

Answer 8

• increase in death rate
• misery (war, disease, famine)

Question 9

What are the preventative controls for population size?

Answer 9

-reuce birth rate

=moral restraint (celibacy, marry later)

=vice (birth control, abortion, infanticide, prostitution, homosexuality)

Question 10

What is happening here in terms of population size and demand?

Answer 10

• the population and resource availability meet
• population size levels off (sigmoid curve) = reaches carrying capacity K

Question 11

What is this equation?

Answer 11

• logistic equation
• r= rate of maximum population growth
• K= carrying capacity (the maximum sustainable population)

Question 12

Why are there the exponential equation as well as the logistic equation?

Answer 12

• exponential= more of a model, says something about the way populations change but mostly theiretical
• logistic= takes into account that population can’t grow forever, thus the carrying capacity is included and fits the experimental data better though the real world not so much (more factors at play there)

Question 13

What are the r-selected species?

Answer 13

• those with:
• small body size
• short lifecycles
• short gestation period
• high fecundity (many offspring)
• resulting high rate of increase

Question 14

What are K selected species?

Answer 14

-those with:

• larger body size
• longer lifecycles
• long gestation period
• low fecundity (not as many offspring)
• resulting in lower rate of increase

Question 15

What does the logistic equation assume?

Was Malthus wrong?

Answer 15

• that everything else remains constant, when in fact it doesn’t that is why in real life the mathematical models are not followed very closely
• sort of wrong, the world has much higher carrying capacity and it changes with incoming technologies etc.