Physics 3: Springs & Waves

Question 1

Simple Harmonic Motion

Answer 1

1. First, it always acts to restore the particle or object to its equilibrium position any time it is displaced. That is, the direction of the restoring force is always toward the equilibrium position.
2. Second, the magnitude of the force is directly proportional to the magnitude of the displacement of the particle or object from its equilibrium position.

Question 2

Springs

Answer 2

F=-kx (Hooke’s Law)

higher k=stiffer spring

Question 3

Oscillating Systems

Answer 3

Question 4

Angular Frequency

ω

Answer 4

• radians per second.
• **ω=2πf=√(k/m)=2π/T **
• where T is the period, 1/f (time to complete one cycle)
• measure of the rate at which the oscillating object would move through an arc of a particular size if the object were traveling around a perfect circle (for which one revolution around the circle equals one cycle).
• dependent on the spring constant and mass attached to the spring but not on the displacement x of the spring.

Question 5

radians

Answer 5

180/π degrees

360 degrees = 2π radians

Question 6

Equation for Position of a spring as it moves through its cycle as a funciton of time

Answer 6

x= X cos (ωt)

• X is the amplitude (maximum displacement from equilibrium), ω is the angular frequency (ω = 2π f = π /T, where T is the period, ,1/f the time to complete one cycle), and t is time*.
• assuming that the spring has maximum displacement at t = 0.

Question 7

Springs as Conservative Systems

(2 conditions, 1 formula)

Answer 7

Conditions: 1) small oscillations, 2) short periods of time

[assume all the potential energy of the spring will be converted to kinetic energy as it oscillates.]

U=(1/2)kx²

K=(1/2)mv²

E=K + U = constant

Question 8

Simple Pendulum

Answer 8

F= -mg sinθ

• where m is the mass attached to the end of the pendulum, g is the acceleration of gravity (9.8 m/s2), and θ is the angle between the pendulum arm and the vertical
• force equation has a negative sign, reminding us that this is a restoring force, always directed in the direction opposite to the displacement and in the direction of the equilibrium position.

Question 9

Angular Frequency of a Pendulum (of length L)

Answer 9

ω= 2πf=√(g/L)

• NOTE: Neither the mass m attached to the pendulum nor the angular displacement θ determines the angular frequency ω
• Can change angular frequency by changing: length or gravity (ie elevator)
• _{<em>Therefore, if two pendulums have the same length, they will demonstrate the same angular frequency irrespective of the masses attached to them or their respective initial displacements. As with two identical springs stretched to different lengths with the same angular frequency but different linear velocities, the two pendulums will be traveling at different velocities.</em>}

Question 10

Pendulums as Conservative Systems

Answer 10

Neglect air resistance and friction and mechanism supporting pendulum above:

• When pendulum is pulled back to its maximum displacement θ, the pendulum has maximum potential energy (U) = mgh
• K=(1/2)mv²
• E=K+U=constant
  *

Question 11

Comparing: mass-spring system and the simple pendulum

Answer 11

Question 12

Frequency and Period

Answer 12

Frequency:

• f=ω/2π
• f=1/T

Period:

• T=2π/ω
• Wavelength*: λ=2π/k
• Speed*: v=fλ

Question 13

Transverse Waves

Answer 13

• sinusoidal waves
• a waveform in which the direction of particle oscillation is perpendicular to the movement (propagation) of the wave
• the particles are oscillating perpendicular to the direction of energy transfer
• ie: Electromagnetic radiation

Question 14

Longitudinal Waves

Answer 14

• sound waves
• particles of the wave oscillate along the direction of travel of the wave motion; that is, the wave particles are oscillating along the direction of energy transfer

Question 15

Displacement (y) of a particle in a wave at a point (x)

Answer 15

y=Y sin (kx - ωt)

where Y is the amplitude (maximum displacement), k is the wavenumber (don’t confuse this k, wavenumber, with the spring constant k in Hooke’s law!), ω is the angular frequency, and t is the time.

Question 16

speed of the wave (v)

Answer 16

v= (fλ) = (ω/k) = (λ/T)

because

v=fλ

k=2π/λ

ω=2πf=2π/T

f=1/T

Question 17

Phase difference

Answer 17

Destructive (vs constructive) interference

Question 18

Traveling Wave

Answer 18

Question 19

standing waves

Answer 19

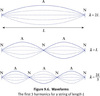
Points in the wave that remain at rest (i.e., points where amplitude is constantly zero) are known as nodes, and points that are midway between the nodes are known as antinodes.

Antinodes are points that fluctuate with maximum amplitude.

The length of the medium dictates the wavelengths of traveling wave that are necessary for establishing the standing wave. Furthermore, the nature of the boundary dictates the appearance of a node or antinode at the boundary itself.

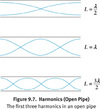
• Closed boundaries are those that do not allow oscillation and that support nodes. The closed end of a pipe and the secured ends of a string are both closed boundaries.
• Open boundaries are those that allow oscillation and support antinodes. The open end of a pipe and the free end of a flag are both open boundaries.

Question 20

Natural Frequency

Answer 20

frequency or frequencies at which an object will vibrate when disturbed

Question 21

forced oscillation

Answer 21

• If a periodically varying force is applied to a system, a system will then be driven at a frequency equal to the frequency of the force
• If frequency of the applied force is close to that of the natural frequency of the system, then the amplitude of oscillation becomes much larger.
• ie: parent pushing a child on a swing

Question 22

Resonance

Answer 22

If the frequency of the periodic force is equal to a natural frequency of the system, then the system is said to be resonating, and the amplitude of the oscillation is at a maximum.

If the oscillating system were frictionless the periodically varying force would continually add energy to the system, and the amplitude would increase indefinitely.

Question 23

Dampening

Answer 23

because no system is completely frictionless, there is always some dampening, which results in a finite amplitude of oscillation.

Question 24

Sound

Answer 24

transmitted by the oscillation of particles along the direction of motion of the sound wave. Therefore, sound waves are longitudinal.

Question 25

speed of sound

Answer 25

• inversely proportional to the square root of density
• directly proportional to the square root of the bulk modulus.
• As a result, sound travels fastest through a solid and slowest through a gas.
• The speed of sound in air at 0° C is 331 m/s.

Question 26

audible waves

Answer 26

frequencies that range from 20 Hz to 20,000 Hz.

• infrasonic waves: Sound waves whose frequencies are below 20 Hz
• ultrasonic waves: frequencies are above 20,000 Hz

Question 27

Intensity of a Sound (I)

Answer 27

I=P/A

• average rate per unit area at which energy is transferred across a perpendicular surface by the wave
• (Thus, Power delievered across a surface such as eardrum: P=IA)
• Proportional to the square of the amplitude **(doubling the amplitude produces a sound wave with 4 times the intensity)
• Intensity is inversely proportional to the square of the distance from the source. (Bc area of a sphere increases as a function of the square of the radius, sound waves transmit their power over larger and larger areas the farther from the source they travel. For example, sound waves that have traveled 2 meters from their source have spread their energy out over a surface area that is four times larger than that for sound waves of the same amplitude and frequency that have traveled 1 meter from their source)

Question 28

Sound Level (β )

Answer 28

• β = 10 log (I/I_o)
• where Io is a reference intensity set at the threshold of hearing, 1 × 10− W/m²
• decibels (dB)

Calculate new sound level:

β_f = β_i+ 10 log (I_f/I_i)

where (I_f/I_i) is the ratio of the final intensity to the initial intensity.

Question 29

Pitch

Answer 29

our perception of frequency

Question 30

Beats

Answer 30

What we hear if the two sound waves have nearly equal frequencies in the audible range, the resultant wave will have periodically increasing and decreasing amplitude,

f_beat = |f₁-f₂|

Question 31

Doppler Effect

Answer 31

difference between the perceived frequency of a sound and its actual frequency when the source of the sound and the sound’s detector are moving relative to each other

• If the source and detector are moving toward each other, the perceived frequency f′ is greater than the actual frequency f,
• if the source and detector are moving away from each other, the **perceived frequency f′ is less than the actual frequency f. **
• f’=f[(v +/-v_D)/ (v-/+ v_s)
• where v is the speed of sound in the medium, v_D is the speed of the detector relative to the medium, and v_S is the speed of the source relative to the medium. The upper sign on v_D and v_S is used when the detector and the source are getting closer together. The lower sign is used when the detector and the source are going farther away from each other.

Question 32

strings (standing waves)

Answer 32

λ=(2L)/n

• where n is a positive nonzero integer (n = 1, 2, 3,… )*
• Pattern: * etc.

From relationship that f=v/λ, the possible frequencies are:

f=(nv)/2L

where n is a positive nonzero integer (n = 1, 2, 3,… ).

Question 33

open pipes

Answer 33

λ=(2L)/n

• where n is a positive nonzero integer (n = 1, 2, 3,… )*
• Pattern:* etc.

From relationship that f=v/λ, the possible frequencies are:

f=(nv)/2L

where n is a positive nonzero integer (n = 1, 2, 3,… ).

Question 34

harmonic series

Answer 34

fundamental frequency( first harmonic): lowest frequency (longest wavelength) of a standing wave that can be supported in a given length of string

second harmonic: frequency of the standing wave given by n = 2 is known as the firstovertone or This standing wave has one-half the wavelength and twice the frequency of the first harmonic. All the possible frequencies that the string can support form its harmonic series.

The waveforms of the first three harmonics for a string of length L are shown.(Note: N stands for node and A stands for antinode.)

Question 35

Closed Pipes

Answer 35

λ=4L/n

where n is odd integers only (n = 1, 3, 5,… ). The frequency of the standing wave in a closed pipe is f=nv/4L